Research Fields: Differential Equations and Dynamical Systems
Quick Links
General Introduction to Differential Equations and Dyanmical Systems
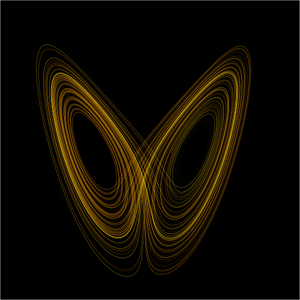
Dynamical systems are used to characterize the changes of natural physical systems, biological systems, and engineering systems with time. These systems usually involve mathematical tools such as ordinary differential equations, partial differential equations and functional differential equations, etc. There are also discrete time dynamical systems and the newly developed lattice dynamical systems.
At present dynamical systems is considered an interdisciplinary science, its origin can be traced back to the mid 17th century, the time when Newton formulated the basic laws of motion and Kepler's law of planetary motion. Hence, dynamical systems and differential equations are originated from physics with a strong tie persist to present days between each of them. For instance, NASA, in its 2001 Genesis Discovery Mission, recruited mathematicians and astrophysicists from JPL Lab at CIT and Europe to design a fuel efficient orbit. This task was accomplished based on the knowledge of classical mechanics and n-body problem, with the aid of mathematical analysis and computers.
Furthermore, ecology, biological mathematics and electric circuit theory owe much of their developments to dynamical systems. Namely, Hodgkin and Huxley claimed their 1963 Nobel honors in Medicine through their research in the cellular signal transmissions. Their work includes the computation of the solution of differential equations and the implications of the solution on biological phenomena. Even the structure of electric circuit can be represented in systems of differential equations. In recent years, several faculty members have made substantial progress in the underlying mathematical theory of cellular neural networks; research on encrypted signal transmissions and synchronization theory too have been actively carried out.
The subjects a la mode like chaos and fractals are closely linked to dynamical systems. Chaos is a natural phenomenon, the understanding and utilization of it would be the pivotal moment of technological development in the next century. A number of the faculties in our department are currently committed to the computation and analysis of chaos including the mathematical model of discrete time dynamical system and lattice dynamical systems, the mechanism of the formation of chaos, and control and synchronization of chaos. Equations of discussion come from electric circuit theory, photonic effects, biological model, fluid mechanics, etc.
TopResearch Interests
Professor Song-Sun Lin (Dynamic system, Zeta function)
|
My research areas are multi-dimensional symbolic dynamic systems and dynamic Zeta function. 1.Multi-dimensional symbolic dynamic system: 2.Dynamic Zeta function: |
Professor Wen-Wei Lin (Numerical Analysis, Matrix Theory and Computation, Optimization, Computational Conformal Geometry)
TopProfessor Chih-Wen Shih (Dynamical systems, Differential equations, Mathematical biology)
TopProfessor Ming-Chia Li (Dynamical Systems, Chaos)
|
In recent years, we study the followings: (1)Coexistence of invariant sets with and without SRB measures in Henon family (2)Topological dynamics for multidimensional perturbations of maps with covering relations and Liapunov condition (3)Positive topological entropy for multidimensional perturbations of topologically crossing homoclinicity (4)Covering relations for coupled map networks (5)Stability of symbolic embeddings for difference equations and their multidimensional perturbations |
Professor Juang Jonq(Coupled Systems, Synchronization, Neural Networks, Neuronal Dynamics, Flocking Problems and Traffic Flow Models)
TopAssociate Professor Jong-Eao Lee (Riemann Surface Theory, Theory of Classical Mathematical Functions, Periodic Solitons Theory of nonlinear evolution equations, and the Applications to the waves)
|
We develop the periodic soliton theory of exactly integrable systems (all are universal nonlinear evolution equations) on Riemann surfaces of genus N, and apply it to generic waves. Three important schemes are involved (each in both theoretical and numerical aspects) : (1) develop the correct complex analysis and techniques of evaluation of path integrals on Riemann surfaces of genus N with various algebraic structures. This is the most fundamental and important tool in doing research waves. (2) study the classical mathematics such as the theory of Elliptic functions, the Theta functions, and the Jacobian Elliptic functions. It is the theoretic foundation to the theory of Riemann surfaces and to the wave theory. (3) Apply the theory and numerical techniques in (a, b) to analyze the exactly integrable systems such as Korteweg-de Vries, sine-Gordon and nonlinear Schrdinger universal partial differential equations which are universal mathematical models for generic waves. The wave theory need much more different mathematical theory to analyze, and is still a wide-open field. Yet, the periodic soliton theory is already successfully applied to generic wave theory sush as water waves(deep, shallow, long, short), laser light, in acoustics, in plasmas, and network signals, etc. |
Associate Professor Shu-Ming Chang (Scientific Computations, Dynamical Systems)
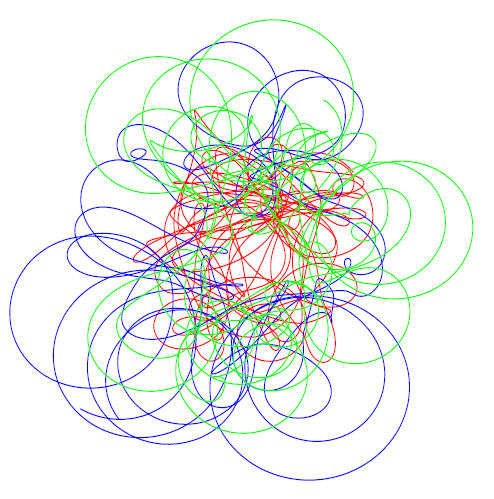
My interest is currently focused on chaotic systems and their applications. We propose a digitalized chaotic map, Variational Logistic Map (VLM), modified from classical logistic maps to be used in secure communication. Compared to a classical logistic map, VLM has large parameter space without windows and can be implemented at low hardware cost. Furthermore, a chaotic crypto scheme, Multi-VLM (MVLM), constructed by four 32-bit VLMs can generate an output sequence with a minimal length equal to 2 to power 128 by a 128-bit external key. At the same time, we study Satake's generalized resource budget model of ecology, that was modified from Isagi's resource budget model. We present mathematical viewpoints and numerical analysis on Satake's generalized resource budget model to rigorously prove that the generalized resource budget model is chaotic in Devaney's sense by using the snapback repeller theory and the topological entropy theory.
TopAssistant Professor Chi-Jer Yu(Differential Equations, Scientific Computation)
TopCourse
Annual Dynamical systems and Differential Equations Courses offered:
- Differential Equations (Sophomore)
- Introduction to Dynamical Systems (Junior, Senior)
- Ordinary Differential Equations (Senior, Graduate)
- Partial Differential Equations (Senior, Graduate)
- Dynamical Systems (Senior, Graduate)
- Chaotic Dynamical Systems (Senior, Graduate)